If a number can be expressed as a product of two whole numbers, then
the whole numbers are called factors of that number.
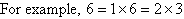
So, the factors of 6 are 1, 2, 3 and 6.
Example 1
Find all factors of 45.
Solution:
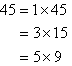
So, the factors of 45 are 1, 3, 5, 9, 15 and 45.
Common Factors
10 = 2 × 5 = 1 × 10
Thus, the factors of 10 are 1, 2, 5 and 10.
15 = 1 × 15 = 3 × 5
Thus, the factors of 15 are 1, 3, 5 and 15.
Clearly, 5 is a factor of both 10 and 15. It is said that 5 is a common
factor of 10 and 15.
Example 2
Find a common factor of:
a. 6 and 8
b. 14 and 21
Solution:
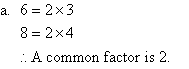
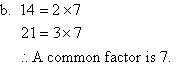
Prime Numbers
If a number has only two different factors, 1 and itself,
then the number is said to be a prime number.
For example, 31 = 1 × 31
31 is a prime number since it has only two different factors.
Note:
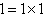
But 1 is not a prime number since it does not have two different
factors.

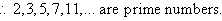
Example 3
Express 150 as a product of prime numbers, i.e. find its prime factor
decomposition.
Solution:

Note:
We try the prime numbers in order of their magnitude.
We observe that:
The prime factor decomposition of a number is unique.
This is called the Fundamental Theorem of Arithmetic. It
provides us with a good reason for defining prime numbers so as to exclude
1. If 1 were a prime, then the prime factor decomposition would lose
its uniqueness. This is because we could multiply by 1 as many times
as we like in the decomposition.
Highest Common Factor
The highest common factor (HCF) of two numbers (or
expressions) is the largest number (or expression) that is a factor of
both.
Consider the highest common factor of 16 and 24.
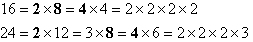
The common factors are 2, 4 and 8. So, the highest common factor
is 8.
Note:
The highest common factor is the product of the common prime
factors.
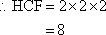
In general:
To find the highest common factor of two (or more) numbers, make prime factors of the numbers and identify the common prime factors.
Then the highest common factor is the product of the common prime factors.
Example 4
Find the highest common factor of 60 and 150.
Solution:
The prime factorisation of 60 is:

The prime factorisation of 150 is:
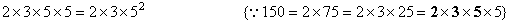

Note:
The highest common factor can also be obtained by a trial and error
method.
For example, the highest common factor of 40 and 45 is 5 because 5 is
the largest number which divides into both 40 and 45 exactly.
Likewise, the highest common factor of 27 and 36 is 9 because 9 is the
largest number which divides into both 27 and 36 exactly.
Key Terms
factors, common factors, prime
numbers, prime factor decomposition, fundamental
theorem of arithmetic, highest common factor, HCF |